The mechanical properties of materials are the properties exhibited by materials when subjected to external forces.
Elasticity#
The ability of a material to not undergo permanent deformation under the action of external forces.
Elastic limit#
$ \sigma_e=\frac{F_e}{A_0} $
Where $ F_e $ is the elastic limit load, with units of $ N $; $ A_0 $ is the original cross-sectional area of the specimen, with units of $ mm^2 $.
Stiffness#
The ability of a material to resist elastic deformation under the action of external forces.
According to Hooke's law:
$ \sigma = E\varepsilon $
Where $ E $ is the elastic modulus, with units of $ MPa $, representing stiffness.
Strength#
The ability of a material to resist deformation and failure under the action of external forces.
Yield strength#
The ability of a material to resist plastic deformation.
$ \sigma_s=\frac{F_s}{A_0} $
Where $ F_s $ is the load at yield of the specimen, with units of $ N $.
0.2% offset yield strength#
For plastic materials without obvious plastic deformation, the stress value at which a permanent deformation of 0.2% is produced is called the 0.2% offset yield strength.
$ \sigma_{0.2} = \frac{F_{0.2}}{A_0} $
Where $ F_{0.2} $ is the load at which a residual plastic deformation of 0.2% is produced, with units of $ N $.
Tensile strength#
The ability of a material to resist being pulled apart under tensile force, and is the maximum stress the material can withstand before fracture.
$ \sigma_b=\frac{F_b}{A_0} $
Where $ F_b $ is the maximum load before fracture of the specimen, with units of $ N $.

Point A is the proportional limit, corresponding to $ \sigma_p $
Point B is the elastic limit, corresponding to $ \sigma_e $
Point C is the upper yield point
Point D is the lower yield point, corresponding to the yield strength $ \sigma_s $
Point E is the start of strain hardening
Point F is the ultimate stress point, corresponding to the tensile strength $ \sigma_b $
Point G is the fracture point, corresponding to $ \sigma_E $
Hardness#
The ability of a material to resist localized deformation, or the ability to resist the penetration of other hard objects into its surface.
Generally, the higher the strength of a material, the higher its hardness.
Brinell hardness HB#
Using a specific load $ F $, a hardened steel ball or hard alloy ball with a diameter of $ D $ is pressed into the surface of the tested material, and after a certain period of time, the load is removed, and the hardness value is determined based on the area of the indentation.
Usually used to test softer materials such as annealed, normalized, tempered steel, cast iron, and non-ferrous metals.

Vickers hardness HV#
Using a diamond indenter with an apex angle of $ 136^\circ $, a four-sided pyramid-shaped indentation is produced on the material surface under the action of load $ F $ (in $ kgf $), and the diagonal length $ d $ (in $ mm $) of the indentation is measured, and the area $ A $ (in $ mm^2 $) of the indentation is calculated. The hardness value is expressed as the value of $ F/A $.
Especially suitable for testing the hardness of very thin layers, such as metal coatings, thin metal sheets, carburized or nitrided parts.

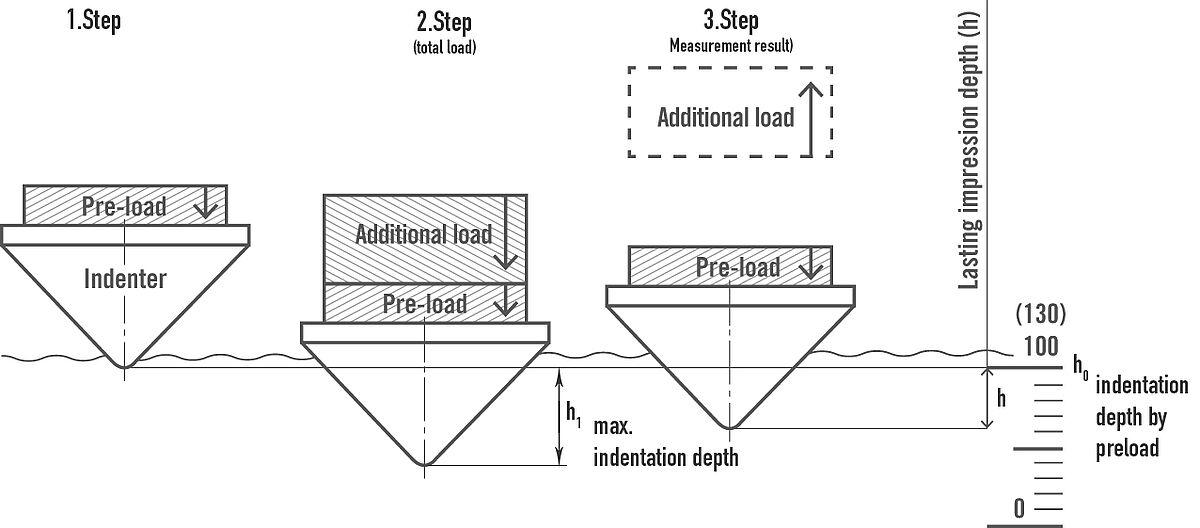
Rockwell hardness HR#
Using a diamond or steel standard indenter with a cone angle of $ 120^\circ $, the indenter is pressed into the surface of the tested material with a specified pressure, and the hardness value is determined based on the depth of the indentation.
Commonly used to test harder materials such as quenched and tempered steel.
Plasticity#
The ability of a material to undergo permanent deformation under stress without fracturing.
Reduction in area#
$ \psi = \frac{A_0-A_1}{A_0}\times 100% $
Where A1 and A0 are the fracture area and the original cross-sectional area of the specimen, with units of $ mm^2 $.
Elongation#
$ \delta = \frac{L_0-L_1}{L_0}\times 100% $
Where L1 and L0 are the length after fracture and the original gauge length of the specimen, with units of $ mm $.
Toughness#
A measure of the energy required for a material to fracture.
Impact toughness#
The ability of a material to resist destruction under impact load. When the specimen fractures, the consumed impact energy $ A_k $, with units of $ J $, is given by:
$ A_k = mg\cdot h_1 - mg\cdot h_2$
The impact toughness value $ a_k $ is the impact energy per unit cross-sectional area at the notch, used to indicate the size of the material's impact toughness, with units of $ J/cm^2 $:
$ a_k = \frac{A_k}{A_0} $
Usually, materials with good plasticity also have higher toughness.
The relationship between the strength, hardness, plasticity, and toughness of a material:
Generally, the higher the strength of a material, the greater its hardness.
Generally, the better the plasticity of a material, the better its toughness.
Generally, as the strength and hardness of a material increase, its plasticity and toughness decrease.
Fatigue strength#
Fatigue#
The phenomenon of fracture occurring in a material under alternating loads below its yield strength.
Fatigue strength#
The maximum stress that a material can withstand under a specified number of alternating loads without fracturing, represented by $ \sigma^{-1} $, commonly known as the fatigue limit.
For steel materials, $ \sigma^{-1} $ is approximately half of its yield strength $ \sigma_b $, while for non-metallic materials, $ \sigma^{-1} $ is usually much lower than that of metals.
Specified number of loads:
Steel materials: $ 10^7 $
Non-ferrous metals and their alloys: $ 10^8 $
This article is also updated on xLog by Mix Space.
The original link is https://ursprung.io/posts/tech/%E6%9D%90%E6%96%99%E7%9A%84%E5%8A%9B%E5%AD%B8%E6%80%A7%E8%83%BD